 首页 » 考研数学 » 高等数学 » 2014年考研数二第17题解析:二重积分、极坐标系
首页 » 考研数学 » 高等数学 » 2014年考研数二第17题解析:二重积分、极坐标系
题目
设平面区域 , , , 计算:
解析
根据题目可知,积分区域 是由两个圆心坐标均为 , 半径分别为 和 的两个同心圆在直角坐标系的第一象限中围成的,如图 01 所示:
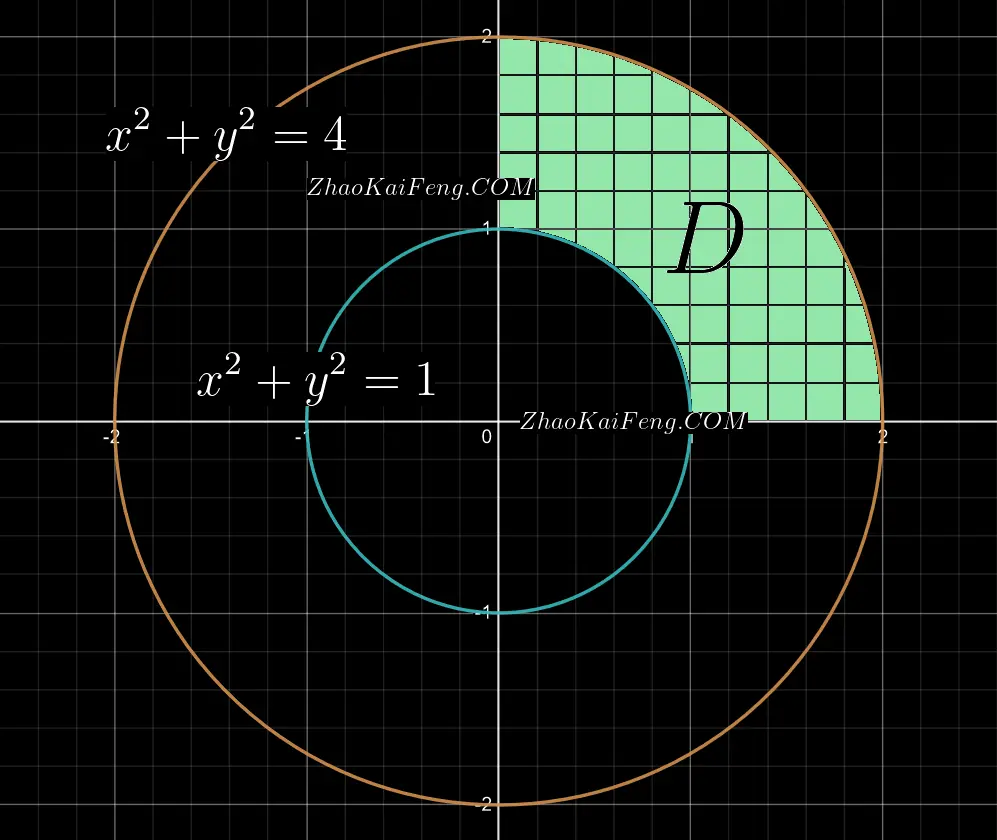 图 01.
图 01.方法一
由于积分区域 是关于直线 对称的,因此,若令:
则:
于是:
其中:
于是:
方法二
其中,由【方法一】可知:
又由于,在 内, 和 的图象是关于直线 对称的,因此:
于是:
于是:
补充
除了上面所示的计算 的方式之外,还可以用如下方式完成对 的求解。
