一、前言 
本文侧重于从考试需要出发对摆线进行说明,摆线相关知识的说明详见:《摆线及其性质》
二、正文 
通常,摆线是通过参数方程表示出来的:
当然,有些题目也可能会把摆线的参数方程写成下面这种形式:
此外,摆线通常是由无数个“拱”组成的,但在考试中,我们一般只研究第一个“拱”,而要形成第一个拱,就需要上面参数方程中的参数
这样的拱的长度是:
拱的高度是:
当然,如果我们忘记了上面的规律,则只需要把
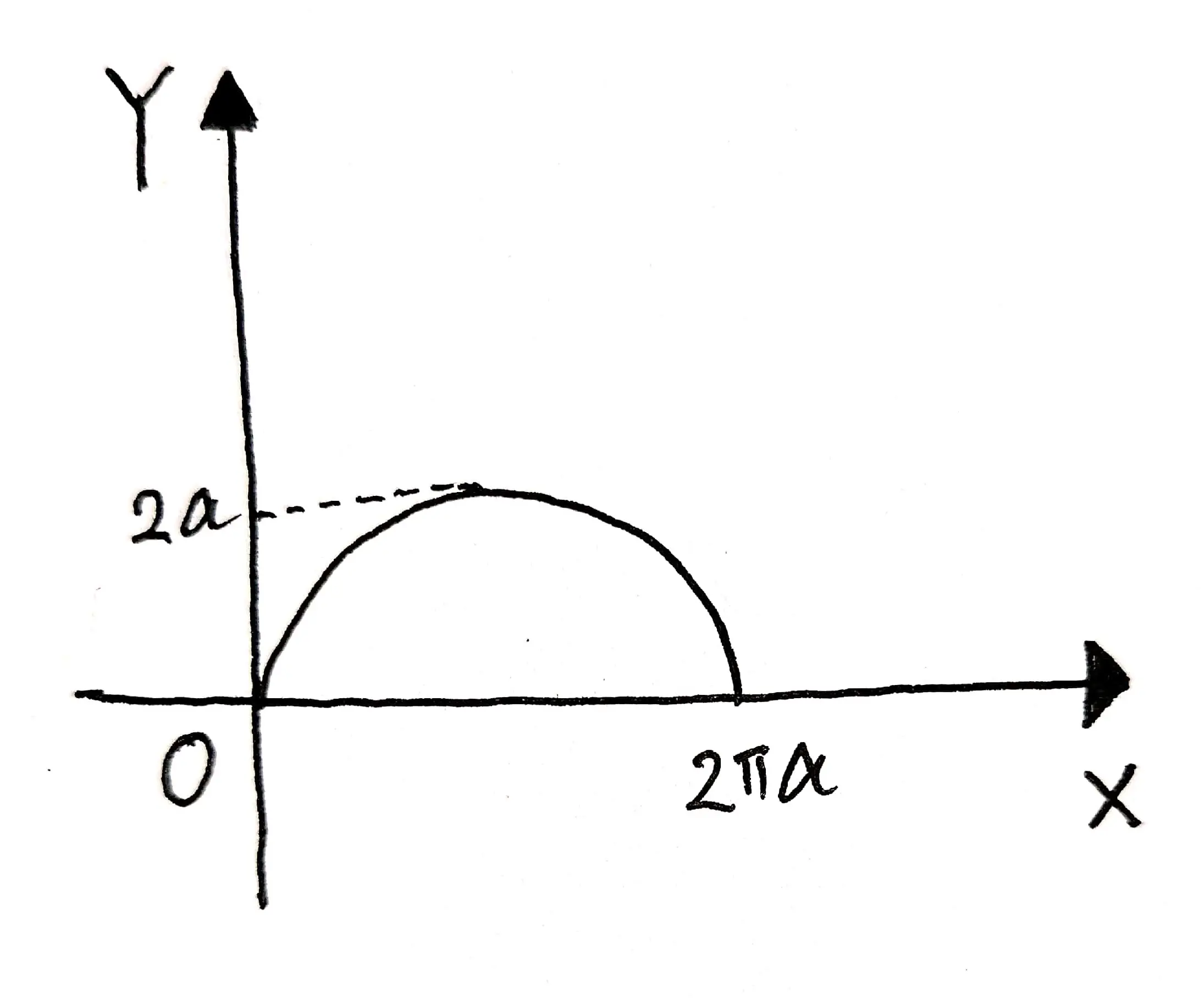
根据上面的分析,我们就可以手绘出摆线的大致示意图:
本文侧重于从考试需要出发对摆线进行说明,摆线相关知识的说明详见:《摆线及其性质》
通常,摆线是通过参数方程表示出来的:
当然,有些题目也可能会把摆线的参数方程写成下面这种形式:
此外,摆线通常是由无数个“拱”组成的,但在考试中,我们一般只研究第一个“拱”,而要形成第一个拱,就需要上面参数方程中的参数
这样的拱的长度是:
拱的高度是:
当然,如果我们忘记了上面的规律,则只需要把
根据上面的分析,我们就可以手绘出摆线的大致示意图:
