题目
已知平面区域
解析
由题可知:
由
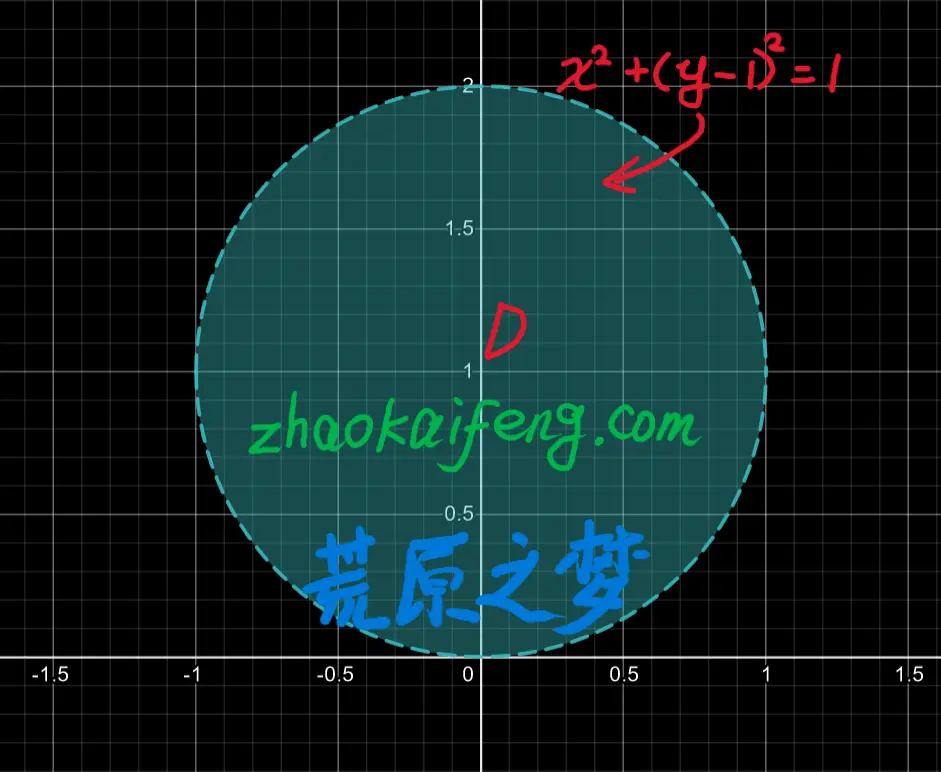
接着:
由于
又:
于是:
于是,我们接下来只需要求解出
根据积分的特点,我们接下来需要将直角坐标系下的二重积分
于是:
接着:
注:
[1]. 根据前面的图 01 可知,变量
的取值范围是: .
注:
[1]. 在区间
上, 和 的图象都是关于 对称的.
于是,由
已知平面区域
由题可知:
由

接着:
由于
又:
于是:
于是,我们接下来只需要求解出
根据积分的特点,我们接下来需要将直角坐标系下的二重积分
于是:
接着:
注:
[1]. 根据前面的图 01 可知,变量
的取值范围是: .
注:
[1]. 在区间
上, 和 的图象都是关于 对称的.
于是,由